Almost-Mathematics
Almost-mathematics is a tool studying $p$-adic Hodge theory. In this section, we use the book of Gabber and Ramero, [20], as our main reference like Peter Scholze in [16].
Unless otherwise stated, all rings are commutative with identity. We fixed a base ring $V$ that contains an ideal $\mathfrak{m}$ satisfying $\mathfrak{m}^{2} = \mathfrak{m}$. And we also assume that $\widetilde{\mathfrak{m}} := \mathfrak{m}\otimes_{V}\mathfrak{m}$ is a flat $V$-module. For the definition of tensor product, see §11.
Question 3.1. Let $(V,\left| \cdot \right|)$ be a non-discrete valuation ring of rank 1, and $\mathfrak{m}$ is an ideal of $V$. If we have $\mathfrak{m}^{2} = \mathfrak{m}$, can we get that $\mathfrak{m}$ is the maximal ideal of $V$?
The basic idea of almost-mathematics is that one neglects $\mathfrak{m}$-torsion everywhere.
Definition 3.2. Let $M$ be a $V$-module. We say that $M$ is almost zero if $\mathfrak{m}M$ = 0. A map of $V$-modules $\phi: M\rightarrow N$ is an almost isomorphism, if Ker $\phi$ and Coker $\phi$ are both almost zero $V$-modules. Then we say that $M$ is almost isomorphic to $N$.
Remark 3.3. A map of $V$-modules $\phi: M\rightarrow N$ is almost injective if $\textrm{Ker }\phi$ is almost zero. And $\phi$ is almost surjective if $\textrm{Coker }\phi$ is almost zero.
Proposition 3.4. A $V$-module $M$ is almost zero if and only if $\mathfrak{m}\otimes_{V} M = 0$.
Proof. Just consider the natural isomorphism $V\otimes_{V}M\cong M$.
Proposition 3.5. A map $\varphi:M\rightarrow N$ of $V$-modules is an almost isomorphism if and only if the induced map $\widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow \widetilde{\mathfrak{m}}\otimes_{V}N$ is an isomorphism.
Proof. First we assume that $\varphi:M\rightarrow N$ is almost isomorphic. Consider the exact sequence $$ \textrm{Ker }\varphi\rightarrow M\rightarrow N\rightarrow \textrm{Coker }\varphi; $$
since $\widetilde{\mathfrak{m}}$ is flat, we have the induced exact sequence $$ \widetilde{\mathfrak{m}}\otimes_{V}\textrm{Ker }\varphi\rightarrow \widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}\textrm{Coker }\varphi. $$
Since Ker $\phi$ and Coker $\phi$ are both almost zero $V$-modules, we have the following exact sequence $$ 0\rightarrow \widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N\rightarrow0, $$
which implies that $\widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N$ is an isomorphism.
Conversely, assume that $\widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N$ is an isomorphism. We consider the exact sequence again $$ \textrm{Ker }\varphi\rightarrow M\rightarrow N\rightarrow \textrm{Coker }\varphi. $$
Then similarly, we have the induced exact sequence $$ \widetilde{\mathfrak{m}}\otimes_{V}\textrm{Ker }\varphi\rightarrow \widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}\textrm{Coker }\varphi.$$
Since $\widetilde{\mathfrak{m}}\otimes_{V}M\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}N$ is an isomorphism, we conclude that $\widetilde{\mathfrak{m}}\otimes_{V}\textrm{Ker }\varphi=\widetilde{\mathfrak{m}}\otimes_{V}\textrm{Coker }\varphi=0$. So we get $\mathfrak{m}\otimes_{V}\textrm{Ker }\varphi=\mathfrak{m}\otimes_{V}\textrm{CoKer }\varphi=0$ as desired.
Lemma 3.6. If $\mathfrak{m}$ is flat, then we have an isomorphism $\widetilde{\mathfrak{m}}\cong\mathfrak{m}$ of $V$-modules.
Example 3.7. If $V$ is a non-discrete valuation ring of rank one, then its maximal ideal $\mathfrak{m}$ is flat as a $V$-module by ([11], Tag0539). Therefore, we have $\widetilde{\mathfrak{m}}\cong\mathfrak{m}$.
Proposition 3.8. The set of all almost isomorphisms forms a multiplicative system.
Proof. First, any identity of $V$-module is almost isomorphic. And the composition of almost isomorphic maps is almost isomorphic. Given a map of $V$-modules $g:X\rightarrow Y$, an almost isomorphic map of $V$-modules $t: X\rightarrow Z$, and a $V$-module $W$. Then we have an induced solid diagram
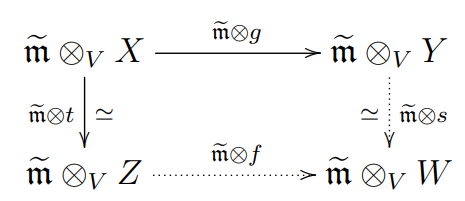
which is made to be commutative with $\widetilde{\mathfrak{m}}\otimes s:\widetilde{\mathfrak{m}}\otimes_{V}Y\cong \widetilde{\mathfrak{m}}\otimes_{V}W$ and $\widetilde{\mathfrak{m}}\otimes f:\widetilde{\mathfrak{m}}\otimes_{V}Z\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}W$. So the solid diagram
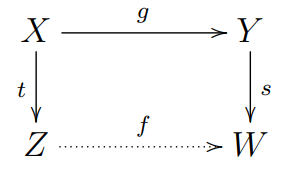
can be made to be commutative with a map of $V$-modules $f: Z\rightarrow W$ and an almost isomorphic map of $V$-modules $s: Y\rightarrow W$.
Given a pair of maps of $V$-modules $f,g: X\rightarrow Y$ and an almost isomorphic map of $V$-modules $t: Z\rightarrow X$ such that $f\circ t=g\circ t$. We have the induced maps $f',g':\widetilde{\mathfrak{m}}\otimes_{V}X\rightarrow\widetilde{\mathfrak{m}}\otimes_{V}Y, t':\widetilde{\mathfrak{m}}\otimes_{V}Z\cong\widetilde{\mathfrak{m}}\otimes_{V}X$ such that $f'\circ t'=g'\circ t'$. Then there exists an almost isomorphic map of $V$-modules $s: Y\rightarrow I$ such that $s':\widetilde{\mathfrak{m}}\otimes_{V}Y\cong\widetilde{\mathfrak{m}}\otimes_{V}I$ satisfies $s'\circ f'=s'\circ g'$ by virtue of $f'\circ t'=g'\circ t'$. Consequently, we have $s\circ f=s\circ g$.
We have just proved the left multiplicative case. However, the right multiplicative case is similar.
参考文献
- [1]HH. Matsumura, Commutative Ring Theory. Vol. 8 of Cambridge Studies in Advanced Mathematics(1989).
- [2]N. Bourbaki, Éléments de mathématique. Fasc. XXX. Algébre commutative. Chapitre 6: Valuations. Actualités Scientifiques et Industrielles, No. 1308. Hermann, Paris, 1964.
- [3]N. Bourbaki, Algebra II, Chapters 4-7, Elements Of Mathematics, Springer, Berlin, Heidelberg, 2003.
- [4]Pierre Antoine Grillet, Abstract Algebra, 2nd ed., Graduate Texts in Mathematics, vol. 242, Springer-Verlag New York, 2007.
- [5]Serge Lang, Algebra, 3nd ed., Graduate Texts in Mathematics, vol. 211, Springer-Verlag New York, 2002.
- [6]Serge Lang, Algebraic Number Theory, 2nd ed., Graduate Texts in Mathematics, vol. 110, Springer, New York, NY, 1994.
- [7]Siegfried Bosch, Lectures on formal and rigid geometry, volume 2105 of Lecture Notes in Mathematics. Springer, Cham, 2014.
- [8]S. Bosch, U. Güntzer, and R. Remmert, Non-Archimedean analysis. A systematic approach to rigid analytic geometry, Grundlehren der mathematischen Wissenschaften 261, Springer-Verlag Berlin, Heidelberg 1984.
- [9]Linus Kramer, Locally Compact Groups and Lie Groups, Part I Basic Properties of Topological Groups, 1 Topological Groups, https://www.uni-muenster.de/AGKramer/content/ch1.pdf, 2020.
- [10]Tammo Tom Dieck, Algebraic Topology, European Mathematical Society, Chapter 1 Topological Spaces, 1.7 Topological Groups, 2008.
- [11]Stack Project authors, Stack Project, https://stacks.math.columbia.edu/, 2022.
- [12]Dinakar Ramakrishnan and Robert J. Valenza, Fourier analysis on number fields, Graduate Texts in Mathematics, vol. 186, Springer-Verlag, New York, 1999.
- [13]Pierre Deligne, Cohomologie Étale, Séminaire de Géométrie Algébrique du Bois-Marie SGA 4 1/2, Springer-Verlag Berlin Heidelberg, Lecture Notes in Mathematics, volume 569, 1977.
- [14]Alexander Grothendieck and Michèle Raynaud, Revêtements Étales et Groupe Fondamental, Lecture Notes in Mathematics, Volume 224, Springer, Berlin, Heidelberg, 1971.
- [15]Alexander Grothendieck, Artin, M. and Verdier, J. L. Théorie des Topos et Cohomologie Étale des Schémas. Séminaire de Géométrie Algébrique du Bois-Marie 1963-1964 (SGA 4), Springer-Verlag Berlin Heidelberg, 1973.
- [16]Peter Scholze, Perfectoid Spaces, IHES Publ. math. 116 (2012), pp. 245-313.
- [17]Peter Scholze, Étale cohomology of diamonds, Preprint, 2018.
- [18]R. Huber. Continuous valuations. Math. Z., 212(3):455-477, 1993.
- [19]Bjorn Poonen, Rational Points on Varieties, Graduate Studies in Mathematics, Volume 186, American Mathematical Society, 2017.
- [20]O. Gabber and L. Ramero. Almost ring theory, volume 1800 of Lecture Notes in Mathematics. Springer-Verlag, Berlin, 2003.
- [21]Ofer Gabber and Lorenzo Ramero, Foundations For Almost Ring Theory, Release 7.5, 2019.
- [22]Jean-Marc Fontaine and Yi Ouyang, Theory of p-adic Galois Representations, preprint, 2008.
- [23]James Dugundji, Topology, Allyn and Bacon, Inc.470 Atlantic Avenue, Boston., 1966.
- [24]Antoine Ducros, Charles Favre and Johannes Nicaise, Berkovich Spaces and Applications, Springer International Publishing, Lecture Notes in Mathematics, Volume 2119, 2015.
- [25]Vladimir G. Berkovich, Spectral Theory and Analytic Geometry over Non-Archimedean Fields, American Mathematical Society, 1990.
- [26]Vladimir G. Berkovich, Étale cohomology for non-Archimedean analytic spaces, Publications Mathématiques de l'Institut des Hautes Études Scientifiques 78, 5-161 (1993). https://doi.org/10.1007/BF02712916.
- [27]Peter J. Hilton and Urs Stammbach, A Course in Homological Algebra, Graduate Texts in Mathematics, Volume 4, Springer-Verlag New York, 1997.
- [28]A. Grothendieck and J. A. Dieudonné, Eléments de Géométrie Algébrique I, Springer-Verlag Berlin Heidelberg New York, 1971.
- [29]A. Grothendieck, Some aspects of homological algebra, https://www.math.mcgill.ca/barr/papers/gk.pdf, 2011.
- [30]Neal Koblitz, Introduction to Elliptic Curves and Modular Forms, 2nd ed., Graduate Texts in Mathematics, Volume 97, Springer-Verlag New York, Inc., 1993.
- [31]Fred Diamond and Jerry Shurman, A First Course in Modular Forms, Graduate Texts in Mathematics, Volume 228, Springer-Verlag New York, 2005.
- [32]Neal Koblitz, p-adic Numbers, p-adic Analysis, and Zeta-Functions, Graduate Texts in Mathematics, Volume 58, Springer, New York, NY, 1984.
- [33]Fernando Q. Gouvêa, p-adic Numbers An Introduction, 3rd ed, Universitext, Springer, Cham, 2020.
- [34]J-P. Serre, A Course in Arithmetic, Graduate Texts in Mathematics, Volume 7, Springer, New York, NY, 1973.
- [35]J-P. Serre, Local Fields, Graduate Texts in Mathematics, Volume 67, Springer, New York, NY, 1979.
- [36]Jean-Pierre Serre, Galois Cohomology, Springer-Verlag Berlin Heidelberg, 1997.
- [37]Tim Browning and Florian Bouyer, Local Fields, Bouyer, https://warwick.ac.uk/fac/sci/maths/people/staff/fbouyer/local_fieldstcc.pdf, 2013.
- [38]Paul J. McCarthy, Algebraic extensions of fields, New York: Dover Publications., 1991.
